Equation Of Parabola Given Focus And Directrix
The vertex of this parabola is.
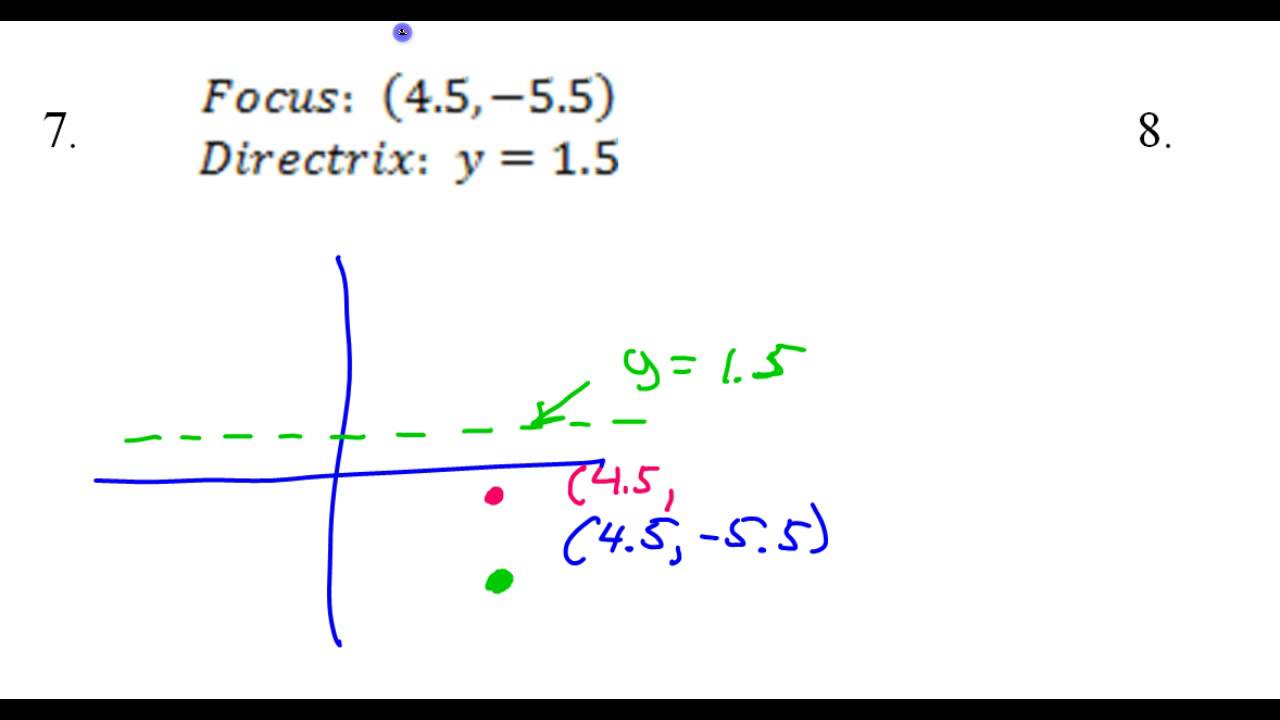
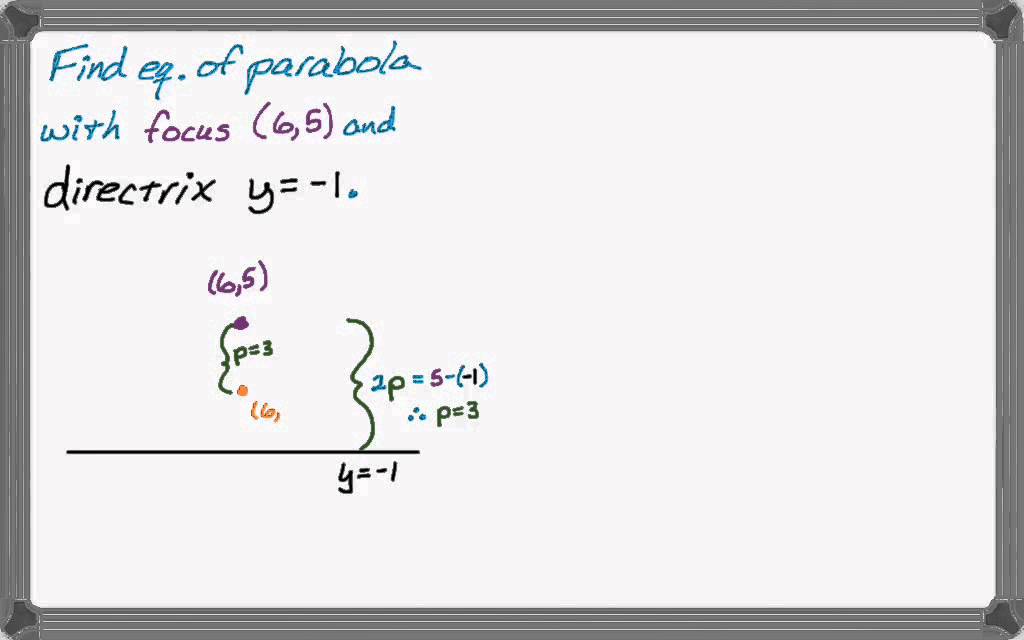
Equation of parabola given focus and directrix. One description of a parabola involves a point the focus and a line the directrix. The focus does not lie on the directrix. The parabola is the locus of points in. Focus and directrix the ellipse and the hyperbola are often defined using two points each of which is called a focus.
The combined distances from these foci is used. Demonstrates how to extract the vertex focus directrix and other information from the equation for a parabola. The word parabola refers to the parallelism of the conic section and the tangent of the conic mantle. Also the parable 1 has been derived from the greek parabole.
In geometry focuses or foci uk. F o k a us. F o s a singular focus are special points with reference to which any of a variety of curves. The curve formed by the set of points in a plane that are all equally distant from both a given line called the directrix and a given point called the focus that.
Work with the equation to find the axis of symmetry focal distance and directrix. To find the axis of symmetry start with the vertex.
Find Equation Of Parabola Given Focus And Directrix 7 2 Youtube
Finding The Equation Of A Parabola Given Focus And Directrix
Day 6 Hw 3 To 8 Write Equation For Parabola Given Focus Vertex Or
How To Determine The Vertex Focus And Directrix Of A Parabola Quora
How To Find Vertex Focus Directrix Of A Parabola
8 2 Graph And Write Equations Of Parabolas Ppt Download
Finding The Directrix Of A Parabola Given Its Vertex And Focus
Equation Of Parabola From Focus And Directrix Youtube
How To Find Vertex Focus Directrix Of A Parabola
Find Focus Directrix Given Equation Youtube
Parabola Equations Mathbitsnotebook Geo Ccss Math
L2d1ii Html
How To Find Vertex Focus And Directrix Of A Parabola
Content Focus Directrix Definition Of The Parabola
Day 7 Hw 1 And 2 Write The Vertex Form Equation For The Parabola
How To Find The Focus Of A Parabola In Standard Form Insaat
Math1 2
Finding The Directrix Of A Parabola Given Its Vertex And Focus
Parabola Focus Vertices Directrix And Latus Rectum
Parabola Complete
Parabola Study Material For Iit Jee Askiitians
Online Tutoring Math English Science Tutoring Sat Psat Gmat
Warm Up 1 08 Identify The Vertex Focus Axis Of Symmetry And
Focus Of A Parabola
Given Vertex Directrix Focus Form Quadratic Equation
Standard Form Of A Parabola Calculator Insaat Mcpgroup Co
Parabola Focus Directrix Review Article Khan Academy
Parabolas With Vertex At The Origin Ck 12 Foundation
Muchlearning 3 2 Parabola
Hw Pg 28 30even Even 50 56even Do Now Take Out Your Pencil
Day 12 Test C 7 To 9 Write The Equation Of The Parabola Given The
Equation Of Parabola Given Focus And Directrix
Parabolas With Vertex At The Origin Ck 12 Foundation
Solution 1 Find The Equation For The Parabola With Focus 3 2 And
Algebra Precalculus How To Derive The Equation Of A Parabola Given
Focus Directrix Of A Parabola From Equation Video Khan Academy
Finding The Directrix Of A Parabola Given Its Vertex And Focus
Parabola Equations Mathbitsnotebook Geo Ccss Math
Find The Equation Of The Parabola Whose Focus Is The Point 2 3 And
Derivation Of The Equation Of A Parabola Math Open Reference
Finding The Vertex Focus And Directrix Of A Parabola Geeksforgeeks
Quiz Worksheet Calculating The Equation Of A Parabola From The
4 The Parabola
Focus Of A Parabola
Focus And Directrix Parabolas Teaching Resources Teachers Pay Teachers
Solved Find The Equation Of The Parabola With The Given F
The Parabola College Algebra
8 2 Graph And Write Equations Of Parabolas Ppt Download
Find Equation Of Parabola Given Focus And Vertex 7 2 Youtube
Parabola Whose Vertex At A Given Point And Axis Is Parallel To Y
